The complex plane

The complex number α =a+bi is completely characterized by the two real numbers a and b. As shown in analytic geometry, a point in a plane is also completely determined by two real numbers, its abscissa and its ordinate. It is therefore possible to establish a one-to-one correspondence between all complex numbers and all points of a plane. If we associate the complex number α =a+bi with the point whose Cartesian coordinates are (a, b), it is clear that to any complex number there corresponds one point of the plane and conversely, to every point of the plane there corresponds one complex number. The points corresponding to real numbers have the ordinate b, and they will therefore be on the horizontal axis. The latter is, for this reason, often referred to as the real axis. Conversely, all points on the real axis correspond to real numbers. Similarly, the vertical axis is the locus of all points corresponding to pure imaginary numbers, and it is therefore called the imaginary axis. The origin of the coordinate system corresponds to the complex number 0.

From what we have said so far it may appear that the association of complex numbers with the points of a plane is merely a kind of visual aid, making it possible to think of complex numbers in geometrical terms. It is, however, much more than that. We shall see that the arithmetic operations correspond to very simple geometric constructions in the plane and that, as a result, the use of geometric language provides not only a very vivid and suggestive terminology for the treatment of complex algebra and analysis, but indeed furnishes in many ways a more adequate expression of the nature of a complex number than the purely arithmetic definition.

The plane whose points represent the complex numbers is called the complex plane. Although there is, of course, a conceptual difference between the complex number α =a+bi and the point (a, b) of the complex plane, we shall often refer to them as though they were one and the same thing. It is convenient to do so, and no confusion can be caused by this practice. Accordingly, rather than refer to the point of the complex plane whose abscissa is Re(α) and whose ordinate is Im(α), we shall speak about the point α.

If instead of the rectangular coordinates a, b we use polar coordinates r, θ in the complex plane, we obtain a different representation of the complex number α =a+bi.

Since a=r cos θ; b=r sin θ,

we have α =r(cos θ + i sin θ), (1)

where the positive number r= 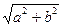is the distance of the point α from the origin. This quantity is called the absolute value, or the modulus of α and is denoted by the symbol 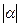. The angle θ is called the argument of α and is denoted by arg α or arg(α). Thus, we have = .

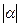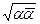If α and β are two complex numbers, we have

|α β |² =α β 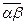= =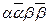|α |² |β |²,

and therefore |α β |=|α ||β |. (2)

If in (2) we replace α by α /β (β ≠ 0) we have | 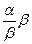|=| ||β | and thus

= .

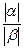By repeated application of (2) we can obtain a result for products of more than two factors. For instance, |α β γ |=|α ||β γ |=|α ||β ||γ | or for n factors α ₁, … α _n

|α ₁…α _n|=|α ₁|…|α _n|.

If in particular α ₁=…=α _n=α, we obtain |α ⁿ|=|α |ⁿ.

As known from elementary geometry, the distance between the points α =a+bi and β =c+di is . Since this is also the absolute value of the complex number α -β, it follows that the distance between the points α and β is |α -β |. It is also known form elementary geometry that the sum of two sides of a triangle is larger that the third side, provided the three vertices of the triangle do not lie on a straight line. Applying this to the triangle (0, α, β) and noting that its sides are |α |, |β |, |α -β |, we find that |α -β |≤ |α |+|β |. If we replace β by – β and observe that |β |=|-β |, we obtain the important inequality

|α +β |≤ |α |+|β |. (3)

The readier will easily verify the sign of equality in (3) will occur if and only of arg α = arg β. If we replace α by α -β, (3) takes the form |α |≤ |α -β |+|β |. If β is again replaced by – β, we thus have the inequality

|α +β |≥ |α |-|β |, (4)

which provides a lower bound for |α +β |. The inequalities (3) and (4) can also be obtained by a direct computation.

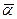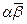We have |α +β |²=(α +β)( + )= |α |²+ + +|β |²= |α |²+2Re(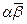)+|β |².

Since α ²≤ α ²+b², the real part of a complex number cannot be larger than its absolute value. Hence, Re(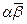)≤ | |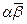=|α ||β |, and it follows that

|α +β |²≤ |α |²+2|α ||β |+|β |²=(|α |+|β |)²,

which is equivalent to (3). Inequality (4) is obtained in a similar fashion if it is observed that Re(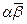)≥ -|α ||β |. It will be a useful exercise of the reader to determine by the use of this method the cases in which the sign of equality holds in (3) and (4).

An elementary geometric argument, combined with the definition of the sum of two complex numbers, shows that the four points 0, α, β, α +β form a parallelogram in which 0 and α +β are opposite vertices. The addition of two complex numbers can therefore be carried out graphically by the parallelogram construction familiar from vector analysis.

*Source: Z.Nehari Introduction to Complex Analysis, p.6-9.

UNIT 4

LINEAR TRANSFORMATION